In high school statistics class, my teacher presented a probability problem that haunts me to this day. It was a puzzle inspired by the TV game show Let’s Make a Deal and named after its longtime host, the late Monty Hall. The setup is simple:
Most people think it doesn’t matter whether they stick with their original choice or switch to the other unopened door because the odds are 50–50—that it’s nothing more than a coin toss. But you should always switch doors. You win two thirds of the time if you switch and one third of the time if you stay. In other words, switching doors doubles your chance of winning.
On supporting science journalism
If you’re enjoying this article, consider supporting our award-winning journalism by subscribing. By purchasing a subscription you are helping to ensure the future of impactful stories about the discoveries and ideas shaping our world today.
This counterintuitive problem, first described in relation to the TV show in 1975, is taught in introductory math and statistics classes across the world. But it was widely popularized in 1990 in Parade magazine. After writer Marilyn vos Savant wrote about the puzzle in her Ask Marilyn column, she received an estimated 10,000 furious letters declaring the answer she gave was wrong, including 1,000 or so that were signed by people with a Ph.D. in their title. The whole affair was so spectacular that it made the front page of the New York Times.
[Play science-inspired games, puzzles and quizzes in our new Games section]
How are the odds not 50–50? Since that fateful column, mathematicians, psychologists and philosophers have been trying to understand what makes this answer uniquely hard to grasp. They’ve found that some of the most common cognitive biases may be to blame, along with a core misunderstanding of how probability works. “Pretty much everybody, even people who are well trained in mathematics, takes the wrong intuitive approach to the Monty Hall dilemma,” says Walter Herbranson, a comparative psychologist at Whitman College. “And that’s [an] indicator that they’ve got a perfectly normally functioning human brain.”
By revisiting the problem again and again over the past decade, I’ve fallen victim to every one of these biases and errors in reasoning. In the end, understanding why the odds are not 50–50 required a mind-bending shift in perspective.
One of the most straightforward ways to see why you should always switch doors is to draw out the possible outcomes. Say you pick door 1. There are three possible realities: the car is behind door 1, 2 or 3.
If the car is behind door 2, Monty Hall will open door 3 and offer for you to switch to door 2. Switching yields the correct door.
If the car is behind door 3, Monty will open door 2 and offer for you to switch to door 3. Switching yields the correct door.
If the car is indeed behind door 1, Monty will open either door 2 or door 3 and offer for you to switch to the one he didn’t open. Switching yields the wrong door.
Put simply, if you selected the correct door initially (which happens 1/3 of the time), you shouldn’t switch when you’re offered the chance. If you chose the wrong door initially (which happens 2/3 of the time), you should switch.
This basic decision tree proves two things: the probability isn’t 50–50, and you’re better off if you always switch. Yet psychological research has shown not only that people usually believe the choice is 50–50 but also that they decide to stick with their original door around 85 to 90 percent of the time. What’s more, they typically continue to choose to stay put even as evidence surmounts that they shouldn’t, says social psychologist John Petrocelli of Wake Forest University. Petrocelli had participants play the Monty Hall game over and over, allowing them to repeatedly observe that the prize is more often behind the “switch” door—but it took many trials for these participants to learn that they should be switching.
He then ran the test with a series of simple coin flips. Imagine you were flipping a coin repeatedly and betting on its outcome each time. How long would it take you to notice that the coin was biased and landed heads-up 2/3 of the time instead of 1/2?
“Most people think they would start to recognize that after about 40 flips. But they don’t,” Petrocelli says. His research has attributed this lack of learning to what he calls “kick-in-the-pants” thinking, or counterfactual thinking. After we make decisions, we often simulate the outcomes of the choices we didn’t make by telling ourselves something like “If only I had chosen differently, I might have won.” Such counterfactuals are not all that salient if you stick with your original door and lose, but they’re extremely salient when you switch and lose—probably because it feels worse to have had and lost than to never have had at all. Those competing alternatives to reality can cloud your memory, Petrocelli says, and “you’re not going to see the pattern that’s emerging before you.”
Even when they learn how the problem works, many people don’t believe they should switch. That’s because the explanation above, while elegant and accurate, isn’t convincing—and not just for people who encountered it as a statistics student, like me. Even prolific mathematician Paul Erdős was tripped up, according to his childhood friend and fellow mathematician Andrew Vázsonyi. In a 1999 article in the Decision Sciences Institute’s publication Decision Line, Vázsonyi described Erdős getting flustered when his friend presented him with the problem for the first time, demanding a reason for why he should switch and then responding with “what’s the matter with you?” when Vázsonyi did not provide one. “I said that I was sorry, but I didn’t have a commonsense explanation,” Vázsonyi recounted.
There is one commonsense explanation that statistics professors will often provide if you tell them you’re confused about the Monty Hall problem. Imagine there are 100 doors instead of three. Only one has a car behind it, and the other 99 have goats. You select a door, say, number 1, and then Monty walks down the line, flinging open door after door. He skips right over number 72, leaving it closed, before opening the rest. Do you want to stick with number 1 or switch to 72? Here you really should switch. Your chance of winning is 99 percent if you do.
“People will usually believe you at that point,” says Jason Rosenhouse, a mathematician at James Madison University, who wrote a book on the Monty Hall problem. “But it doesn’t quite get at the mathematical issue” at the heart of the problem.
He’s right: after hearing the 100 doors explanation, I believed the correct choice was to switch—but only reluctantly. The idea that the chances are 50–50 has a sort of gravity that I felt I couldn’t escape. I was constantly getting snagged on one baffling question: Why does my first choice among the three doors seem to “affect” my second choice between switching and sticking? My gut intuition was that the probability should reset between the two choices, with the second having nothing to do with the first.
“That [assumption] is so natural, and that seems so obvious, but it’s actually wrong,” Rosenhouse says. That’s because Monty Hall knows what door the car is behind, and he chooses his “tease reveal” door accordingly. If he didn’t do this and instead just opened one of the two unselected doors at random—giving him a genuine chance of accidentally revealing the car—then your “stick or switch” choice would indeed be 50–50. But Monty’s knowledge and constraints change everything. To fully understand why this is true, you need to step into Monty’s shoes.
Last year, as I was explaining my love-hate relationship with this problem to my friend and her father—who teaches military strategy and game theory—we decided to set up the game so we could play it in person. I placed a peanut M&M under one of three cups and assumed the role of Monty Hall. After 10 years of intermittent frustration, everything finally clicked.
Try stepping into Monty’s shoes for yourself:
Let’s play again. This time, you are the host.
You know what’s behind each door,
but the player doesn’t.
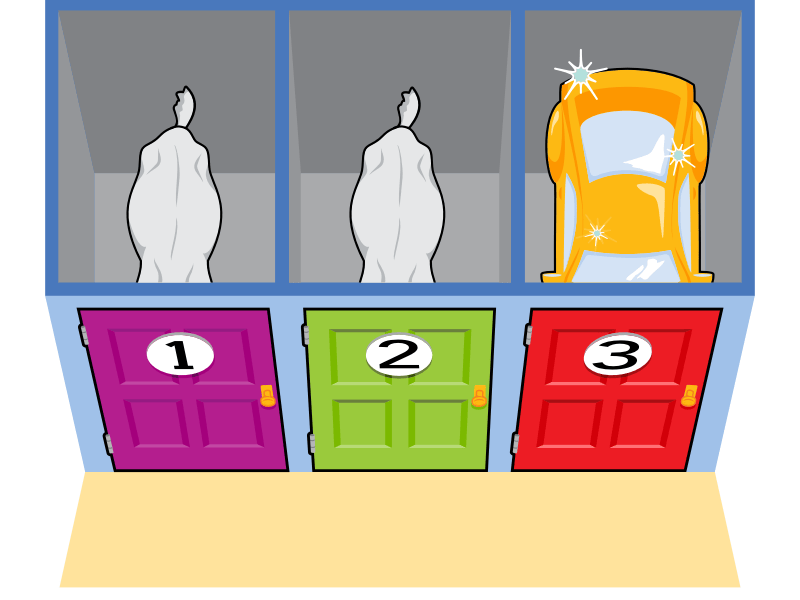
You ask the player to pick a door.
Occasionally—one out of three times, on average—the player will randomly pick a door hiding a car as their
first choice. In this round, that’s door three.
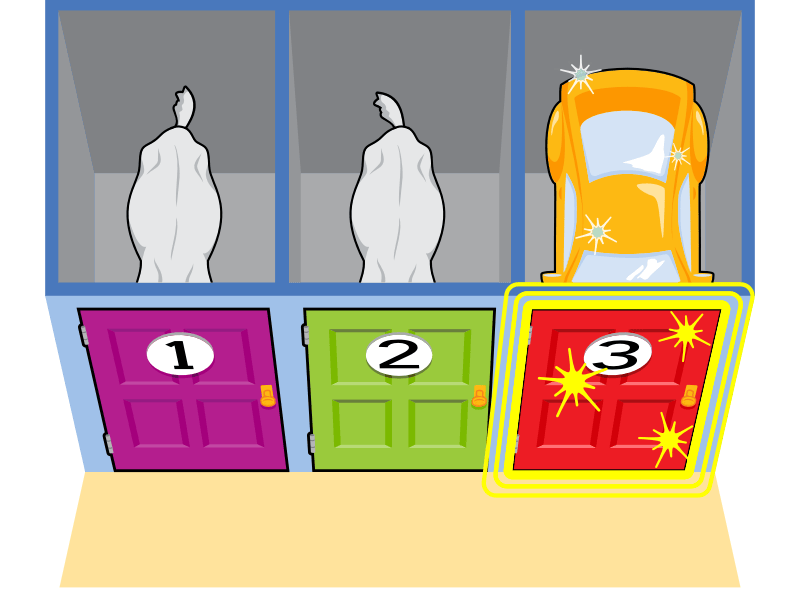
As host, you can then open either of the other two doors because they both hide goats. (The host can only
choose to reveal goats.)
You throw open door two,
revealing a goat.
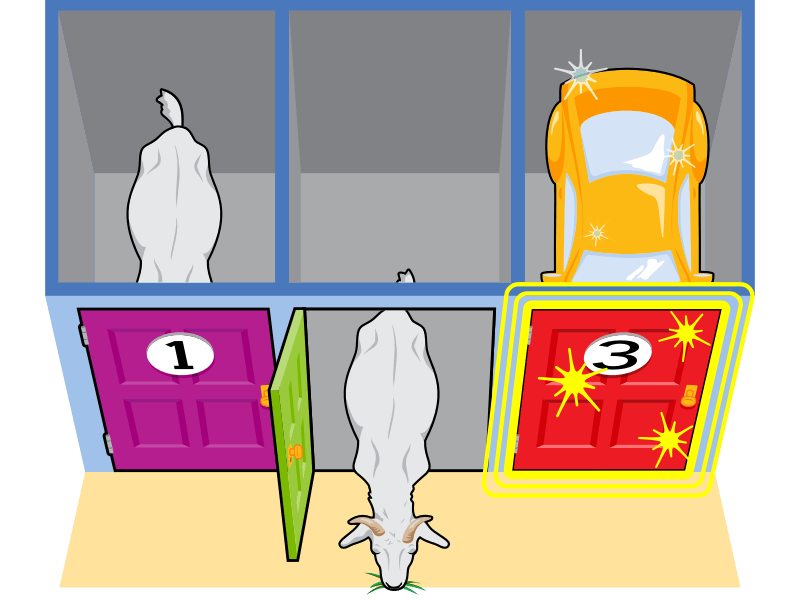
Then you give the player the option of switching their selection to door one.
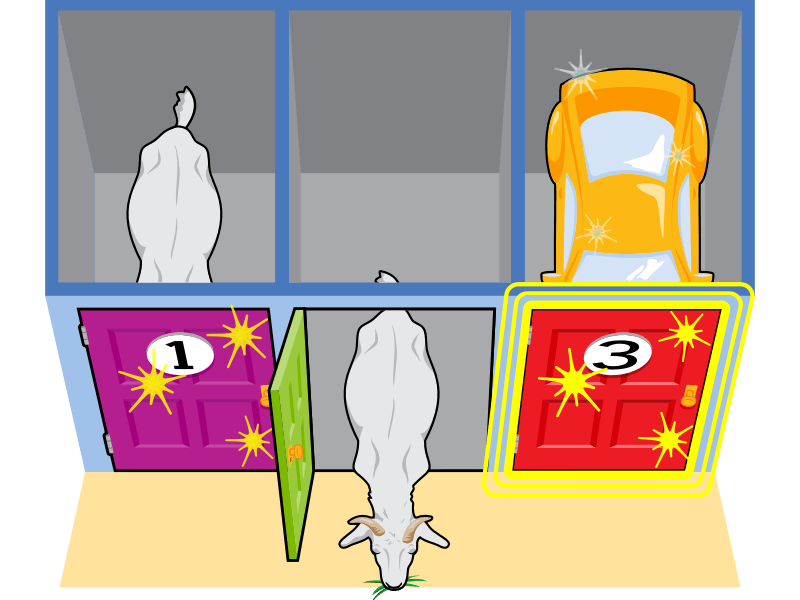
In this scenario, the player will win if they remain with their original choice, door three.
But more often—two out of three times—the player will pick a door hiding a goat as their first choice.
Let’s reset the game. In this round, the player starts by choosing door one.
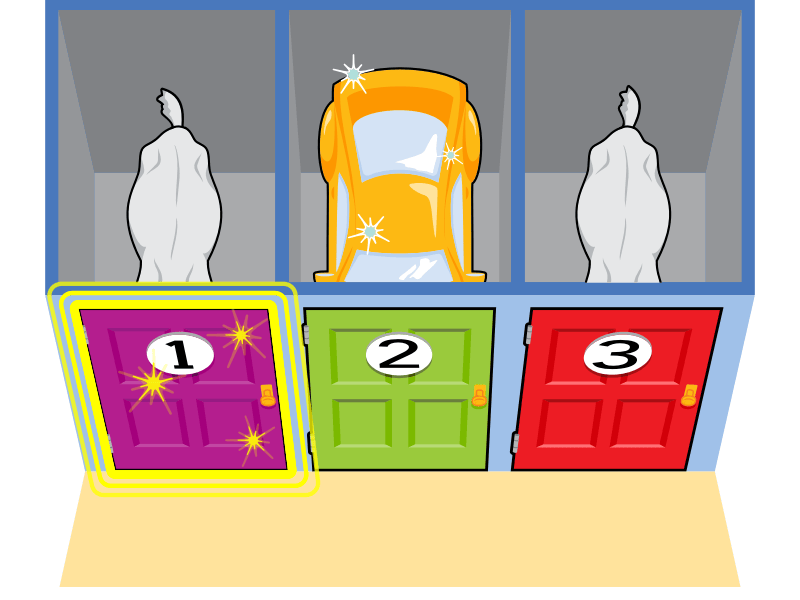
As host, you must now open one of the other two doors.
You can’t choose the car.
Thus, your only option as host is door three.
You open door three and reveal a goat.

Now you give the player the option to remain with door one or switch to door two.

If they stay, they lose.
If they switch, they win.
So, yes, even though one in three times, the player might choose the car first and benefit from not
changing their mind …
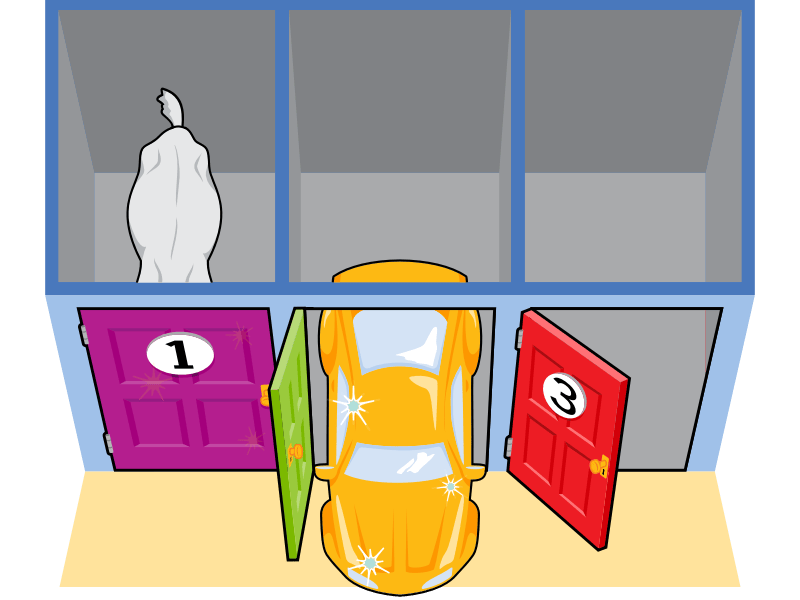
…statistically speaking, it’s in the player’s best interest to always switch their door choice.
Shifting from playing the contestant to playing the host helps many people understand why they should switch doors. In a 2003 study researchers found that people who played the game from Monty’s perspective were more likely to say that game show participants should switch doors than those that played as the participant. (They also had to be prompted to think through what would happen if the participant had picked each of the three doors.) Petrocelli and his colleagues had the same experience with their own research. Programming the problem as part of their study made it “crystal clear” why switching has such an advantage, he says.
Here’s what you can see when you play as the host: Monty Hall isn’t some mysterious figure making shadowy decisions to manipulate, deceive or otherwise trick the contestant. In fact, Monty makes practically no meaningful decisions at all—to the point that playing as the host is practically boring. In two out of three games, the participant picks a goat the first time, meaning the host doesn’t have a choice in what door they open to tease them. In one out of three games, the participant picks the car the first time, so the host picks between the two goat doors at random for the tease reveal.
While the tease reveal feels like new and important information to the contestant, Monty sees that it is simply a distraction. The host is telling the contestant something they already know: “Hey, one of these two doors definitely doesn’t have the car.” As Monty, you see that by opening a goat door, you aren’t giving them information—you’re giving them an opportunity.
That opportunity is two doors’ probability for the price of one. In a game like this, you can’t pick two doors. But that is effectively what the contestant is doing when they decide to switch.
Imagine it like this: You’re the contestant, and you pick door 3. Then the host asks if you want to switch to both doors 1 and 2. Heck, yeah—of course you’re going to take two doors if that’s an option. Then the host opens door 1 and reveals a goat. Do you still want to stick with both doors 1 and 2 or switch to just door 3? You stick with doors 1 and 2 because nothing has changed. You already knew one of your two doors contained a goat. That’s old news. But you’ve still got a 2/3 chance that your selection contains the winning door. The fact that one of your two doors was opened and all of your hopes now rest in the other is completely irrelevant.
When the tease-reveal door is opened, “people think that everything has changed, but the situation is the same as in the beginning,” says Elisabet Tubau, a psychologist at the University of Barcelona. That “is the illusion in the problem.”
“It’s almost like a magic trick, right?” says Christopher Pynes, a philosopher who studies logic and the philosophy of science at Western Illinois University. You may see two possible doors in front of you, but that’s just some clever sleight of hand. What you’re really betting on is not one door versus the other but the probability that you were right the first time versus the probability that you weren’t. You’re being given the chance to bet against your original choice, with the probability of two doors collapsed into one thanks to the throwaway tease reveal.
Here the Monty Hall dilemma is preying on something called the equiprobability bias: we assume that all presented outcomes are equally likely. In an exaggerated form, it’s like rolling a die and asking someone what the odds are that you rolled a one or “not a one,” explains Patrick Onghena, who studies scientific methodology and statistics at the university KU Leuven in Belgium. If you didn’t understand how dice worked, you might incorrectly think that the odds are 50–50 because there are two options being presented to you. But once you understand that a die has six sides, you see that these two choices aren’t equivalent.
Though I’ve now achieved Monty Hall enlightenment, thinking about the problem in the “correct” way still feels like bending my mind out of shape. I keep stubbornly returning to that one question: Why can’t the probabilities just reset once there are only two doors left?
When I ask Pynes about this, his answer reveals a misunderstanding I’ve had about probability for my whole life. “Imagine you turned on the television [during the middle of this game] and there are only two doors. You don’t know anything else, and you pick a door,” he says. “That’s a true 50–50 choice. You didn’t have the option of the third one because you’re coming into the situation after.”
But unlike you, the game show contestant still has a 2/3 probability of getting it right if they switch. That’s because the probabilities we have been talking about this entire time are not tied to the doors and the car and the goats but to the observer. Probability, in other words, is in the eye of the beholder.
This more subjective way of thinking about probability is at the heart of so-called Bayesian reasoning. Reverend Thomas Bayes, a theologian who lived during the 1700s, thought about probability in terms of degrees of certainty based on evidence. His theorem can take prior knowledge of the probability that an event will occur and update it when new information comes to light. This approach to statistics is centered around a person making an estimate—and what that person knows about the likelihoods of different outcomes.
Today Bayes’s theorem is used to test vaccines, map the cosmos and train machine-learning algorithms. But it is not the default way that scientists and mathematicians approach questions of probability. That honor goes to something called frequentist reasoning, which treats probability more like a physical property that can be revealed through repeated tests or simulations. Is this coin biased? The frequentist flips it many times to find out. Should I switch doors or stay put? The frequentist runs many computer simulations and sees that the car is behind the “switch” door 2/3 of the time. Here the probability of these events is treated as a physical thing, a ground truth to be discovered.
Unfortunately, this approach does little to reveal the nuances of the Monty Hall dilemma. A frequentist, looking at the game show stage, might attach probability to the doors and the car and not to the participant doing the guessing. “My hypothesis,” Vázsonyi said in The Man Who Loved Only Numbers, by Paul Hoffman, “is that Erdős had this idea of probability as being attached to physical things and that’s why he couldn’t understand why it made sense to switch doors.”
When I ask vos Savant, the writer of the 1990 Parade column, what she thinks the enduring lesson of the Monty Hall dilemma is, her answer doesn’t involve competing theories in statistics. The puzzle, she says, is about humility. “It promotes a dandy demonstration of a human frailty,” vos Savant explains, “the disbelief that we could be wrong and the tenacity, sometimes aggrieved, with which we hold our earlier judgments, especially when we feel certain.”
